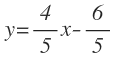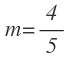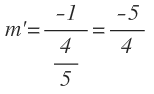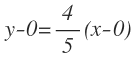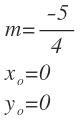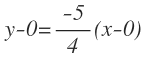Would you like to know how to calculate the equation of a line? What data do you need to calculate it? How to interpret the equation of a line?
One of the biggest problems you usually have in finding the line equation is that most of the time it is not clear what form this equation takes. Therefore, it is not known what each of the terms of the equation means, much less how to calculate it.
That’s why I’m going to explain a very simple way to calculate the equation of a line and what data you need as a minimum.
There are other ways of calculating the equation of a line, but what I am going to teach you next will serve as a starting point and will get you out of more than one predicament. For me it is the easiest way to calculate the equation of a line and you will also be able to solve all the exercises that you need to calculate lines, except those that tell you to calculate a certain form of equation.
Once you assimilate it, you will be able to obtain other forms of the equation of a line more easily, but we’ll leave that for later.
I’m going to start by explaining what the equation of a line is like
How is the line equation? The explicit line equation
The equation of a line can have many forms. But now, let’s focus on this one:
This is the explicit equation of a line. In the first member we have the clear y and in the second member we have two terms, one with x multiplied by the coefficient m and another term formed by the coefficient n.
What do coefficients m and n mean?
I’m going to explain briefly what each one of them means. I don’t want to go into detail because I would give for another whole article and that’s not the purpose of this post either. I just want you to have a global vision.
m is the slope. The slope has to do with the inclination of the line with respect to the x-axis. The steeper the slope, the more inclined the line and the less inclined the slope. It can be positive or negative.
n is the point where the line intersects the y axis:
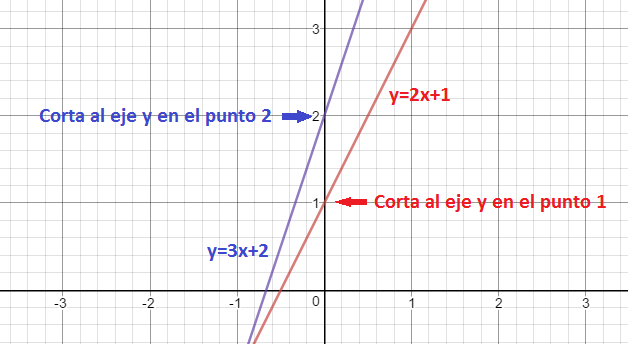
For example, these are two equations of two lines:
If we represent them, we have:
The first line:
Has a slope is m=2 and cuts to the y-axis at point 1, as n=1
The second line:
Your slope is m=3, so it is more inclined than the previous one. On the other hand, n=2, so this line cuts to the axis and at point 2.
How to calculate the slope of a line?
There are many ways to calculate the slope of a line. I’m going to show you some of them, which is going to be the most used.
As mentioned before, the slope indicates the inclination of the line with respect to the x-axis. This slope is calculated by dividing the vertical distance by the horizontal distance between two points on a line.
Those two points of a line, in general will have coordinates (x1,y1) and (x2,y2) for points 1 and 2 respectively:
The vertical distance is calculated by subtracting the y-axis coordinates from each point, and the horizontal distance by subtracting the x-axis coordinates from each point. Therefore m can be calculated with this formula:
On the other hand, the line forms an angle α with the horizontal. Another way to calculate the slope, if this angle is known is with the tangent:
Parallel and perpendicular lines.
Another way to obtain the slope of a line is to indicate that it is parallel or perpendicular to another given line.
Siendo m’ the slope of the perpendicular line.
Therefore, the first thing to do in these cases is to calculate the slope of the given line. To do this, we must put it in its explicit form:
Y the quotient m, will be the slope of the line, that is, the number that is multiplied by x. I remind you that if it has nothing, it is equivalent to having a 1.
For example, what’s the slope of this line?
To obtain it, we first leave the equation in its explicit form:
And now it is clearer that the slope is:
What slope would another line have parallel to this one?
Any line parallel to the previous one will have the same slope, i.e. the same coefficient m:
What slope would another perpendicular line have?
Once we have defined the slope of the given line, the slope of the perpendicular line, keeps this relation as we have seen before:
Thus the slope of the perpendicular line will be:
How to find the equation of a line. Formula of the equation point slope
Well, we already know how to calculate slope, but slope alone is not enough to calculate the equation of a line.
Rectas with the same slope are infinite. Therefore, to define the line we want exactly, we also need to know a point where the line we want to calculate passes.
Therefore, to calculate the equation of a line we need to know the slope and a point through which it passes. With these two data, you can calculate the equation of a line with this formula, known as the slope point equation:
Where m is the slope and X0 e Yo are the coordinates of the point through which the line passes.
For example: Calculate the equation of a line whose slope is m=3 and passes through point P(2,1)
The data that the statement gives us are these:
We replace the data in the dot pending equation:
We Operate:
And finally there’s the equation of the line you’re not asking for
Let’s see another example: Calculate the equation of the parallel line and another perpendicular to it and passing through point P(0,0):
First of all, you have to get the slope. We already got it in the previous example. Its slope is:
We are going to calculate the line parallel to it and passing through point P(0,0), with the formula of the equation point pending:
We replace the data we have:
We are left:
Y we operate to obtain the equation of the line parallel to the given one and passing through point P(0,0):
Now we are going to calculate the equation of the perpendicular line. Its slope is:
We therefore have these data:
That we substitute in the formula:
And finally we have: